Analysis of Laterally Loaded Piles Using Analytical And Numerical Methods
M.R. Motahari1 * and M.R. Maktabipour1
1
Department of Civil Engineering,
Islamic Azad University,
Khomein Branch,
Khomein,
Iran
DOI: http://dx.doi.org/10.12944/CWE.10.Special-Issue1.124
Different methods are presented for the calculation of lateral displacement of a pile under a lateral load. These methods are experimental, and each of them according to simplifying assumptions which they consider, predicts the behavior of the pile with a little approximation. Different methods have been presented to achieve this goal, this paper investigates difference equation, non-dimensional, Broms, Poulos, and the direct methods, and by solving a problem using these methods, as well as using numerical analysis, the results will be compared. The software intended for numerical analysis of the problem is Plaxis 3D software.
Copy the following to cite this article:
Motahari M. R, Maktabipour M. R. Analysis of Laterally Loaded Piles Using Analytical And Numerical Methods. Special Issue of Curr World Environ 2015;10(Special Issue May 2015). DOI:http://dx.doi.org/10.12944/CWE.10.Special-Issue1.124
Copy the following to cite this URL:
Motahari M. R, Maktabipour M. R. Analysis of Laterally Loaded Piles Using Analytical And Numerical Methods. Special Issue of Curr World Environ 2015;10(Special Issue May 2015). Available from: http://www.cwejournal.org/?p=11484
Download article (pdf)
Citation Manager
Publish History
Select type of program for download
| Endnote EndNote format (Mac & Win) | |
| Reference Manager Ris format (Win only) | |
| Procite Ris format (Win only) | |
| Medlars Format | |
| RefWorks Format RefWorks format (Mac & Win) | |
| BibTex Format BibTex format (Mac & Win) |
Article Publishing History
| Received: | 2015-03-21 |
|---|---|
| Accepted: | 2015-03-25 |
Introduction
The ultimate strength of a vertical pile under a lateral load and its displacement are among complex issues in foundation engineering, and one of the issues discussed in soil-structure interaction. In fact, a pile which is almost a rigid member interacts with the soil which shows an elastic or plastic behavior in different ranges of displacement (Broms, B. 1964a).
The methods of determining the ultimate strength of a pile under a lateral load and its displacement are associated with a lot of assumptions and simplifications, so that a simple method can be presented for a complex problem. Each case has to be examined, considering the limited simplifications and assumptions and the validity range of the method (Broms, B. 1964a) and (Palmer, L.A. and Thompson, J.B. 1948).
Piles under lateral loads are generally indeterminate structures, whose analytical solution is not possible, and either approximate methods, which are associated with simplification, or numerical methods have to be used for solution. The more complex a pile and soil interaction model is, the more complex numerical methods are required to use (Murthy, 2007).
Analytical Methods
Analytical models use mathematical language to describe an object. Any analytical model is in fact a theoretical structure which expresses a system with a series of variables and logical and quantitative relationships between variables. Quite extensive researches have been performed and are being done, on the resistance of piles against lateral loads, but given the complexities of the problem, no simple solution or method, which is applicable for all types of soils or piles is provided yet. Many factors affect the strength of a pile under a lateral load (Reese, L.C. and Matlock, H. 1956), (Vesic, AS, 1963), (Vesic, AS 1963).
The most important factor, is the stiffness of a pile, which not only affects the deformations of the pile, but also determines how the failure mechanism of the pile is, whether the failure mechanism occurs due to a rotation in a short member, or will be due to bending as occurs in a long pile (Vesic, AS 1964).
Also the type of loading affects the way a pile resists. Permanent loads (such as a load proceeding from the lateral pressure of a retaining structure) or pulsating loads (such as the loads of rotational machineries) or impact loads (such as loads proceeding from the traffic on the piles of a bridge), affect the way the soil shear strength will be mobilized. Other external factors such as scour in the upper parts of the soil around a pile, or seasonal volume changes in the clay soils also affect the soil strength (Vesic, AS, 1967), (Vesic, AS 1970).
Numerical Modeling
Numerical modeling such as finite element models, has been used extensively to solve a wide range of problems. The main objective of numerical models is in fact solving the governing equations of a problem, such as equations of stability, motion or energy.
Numerical methods do not mainly have a lot of simplifying assumptions. But on the other hand, these methods have computational errors, but in many cases, these errors are much smaller compared to the simplification errors in analytical methods. Since numerical methods solve the governing differential equation of a problem for nodes, generally by dividing this network into smaller parts and increasing the number of nodes, the accuracy of the calculations can be increased. But this takes more time and money to solve a problem. Nowadays, due to the growth of technology, and the significant increase in computational speed, because of the existence of computers with powerful processors, the use of numerical methods, as the main solution to solve the governing equations of engineering problems, is more than ever taken into account, and the use of software programs with numerical solution algorithms has become a necessity, due to the specific advantages of these methods, in achieving higher accuracy, and spending less time to achieve results. Due to the extent of the problems and complexities of any physics problem, numerous numerical methods have been devised and developed, among which finite element, finite difference, boundary element, and discrete element methods can be noted, each with their own specific characteristics.
This article introduces the different methods of analysis of a pile under a lateral load (5 different methods), and by solving a sample problem, by using analytical methods including difference equation, non-dimensional, Broms, Poulos, and the direct methods and also numerical modeling, values of displacement made in the head of the pile under a lateral load will be calculated.
Calculating the Ultimate Strength of a Pile under a Lateral Load
There are several methods to solve the problems of a pile under a lateral load, each of them have a number of advantages and disadvantages. Some of the methods which are described here, are as follows:
- Difference equation method
- Non-dimensional method
- Broms method
- Poulos method
- The direct method
Organized Difference Equations to Solve A Free-Head Pile Under A Lateral Load
Glaser presented a method for simultaneous solution of these equations, in 1953. In this method, by solving the equations, of the bottom of a pile, and eliminating the variables, the progress will be made towards the top (Figure 1).
 |
Figure 1: Analysis of a pile under a lateral load, using the finite difference numerical method, a- Shows how the deformation of the pile is, b- How to divide the pile into small parts Click here to View figure |
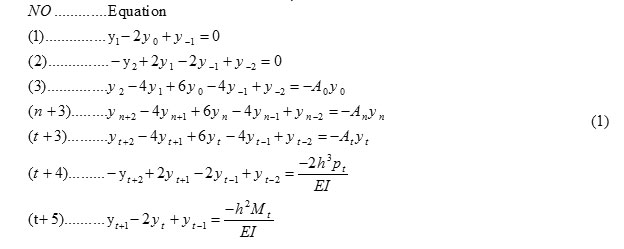
Solution: Considering the relations 1, 2 and 3:
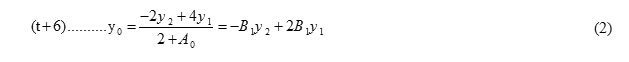
Obtained from Equation 2 and Relation 4
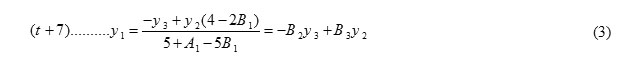
Obtained from Equation 2 & 3 and Relation 5
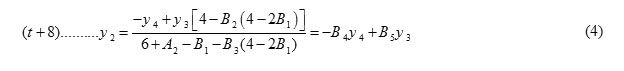
And then

To obtain the displacements, replace the value of obtained from relation t + 11, in relation t + 12, to obtain . Replace these values in relation t + 9, to obtain , etc. Until , and are obtained.
The constants is calculated through the following relations:
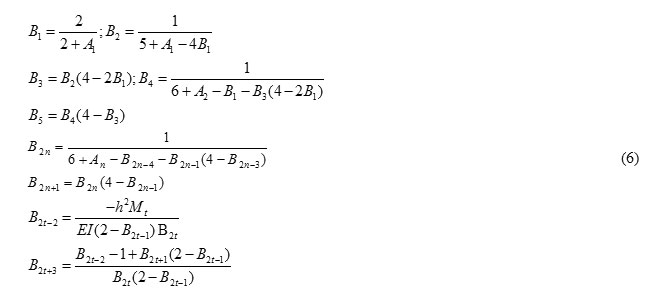
Analyzing A Pile Under A Lateral Load Using the Non-Dimensional Method
The analysis of a pile under a lateral load using the non-dimensional method, which is described in this section, is based on an article published by Reese and Matlock in 1956. The principle of dimensional analysis is used to obtain non-dimensional relations for a pile under a lateral load. Using a theoretical model, the necessary relationships between a sample with optional dimensions and a similar model which is available for solution, is determined.
In long piles, because zero displacements may occur in a greater part of the length of a pile, the pile length loses its efficiency. Thus it has to be replaced by other longitudinal features. For this purpose the stiffness factor T is used, which establishes the relationship between the stiffness of the soil and the bending stiffness of a pile. In which case the displacement of a pile is a function of stiffness factor and the following items:

If it is assumed that the behavior of the pile is elastic, and the amount of displacement of the pile is small, the principle of superposition can be applied, and it will be as follows:

Where yA is the displacement of Pv, and yB is the displacement of Mt (Fig. 2).
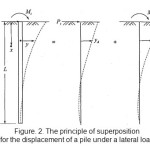 |
|
The displacements for the two intended types are defined as follows:
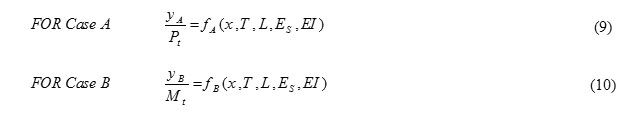
In these equations, fA and fB represent two different functions of same terms. There are 6 terms, and two dimensions (force and length) for the both cases. Therefore, there are four dimensionless parameters, which are described below:
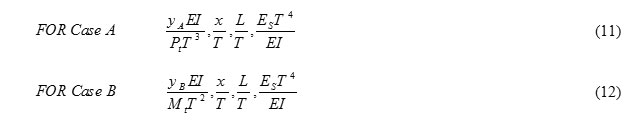
In order to satisfy the similarity conditions, any of these four groups of dimensionless parameters has to be equalized, corresponding to the sample and model. A group of dimensionless parameters, which have a numerical value similar to the model and sample, are defined as follows.
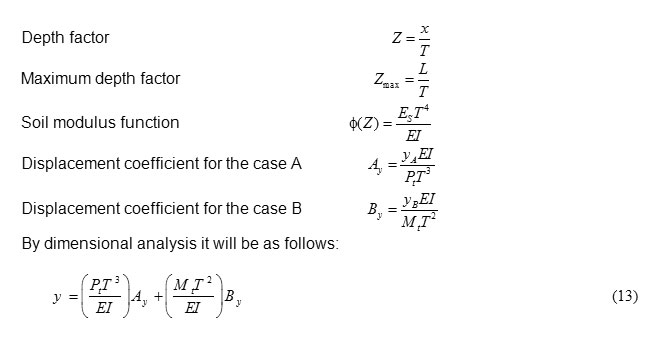
When the head of a pile is free against the rotation, the equation 18 is applicable for calculating the value of displacement. Nonetheless, we more need the value of displacement at ground level. The corresponding equation for this value is:

Analyzing a Pile Using Broms Method
Broms's theory for analyzing the piles, which is explained in this section, is based on articles published by him in 1964.
Broms has provided formulas for calculating the displacement at ground level for granular soils, and has also provided the curves of Figure 3. The vertical axis of this curve is the dimensionless parameter yg (EI)3/5 (nh)2/5 /PtL and its horizontal axis is ηL. This curve is prepared for different values of e/L.

 |
|
If ηL<2 the pile has a rigid behavior (short pile), and ηL<2 if the pile has a non-rigid behavior (long pile). As it can be seen in the curve of Figure 5-14, for ηL<2 , changes in the pile length have a great impact on the displacement at ground level, while for ηL<2, changes in the pile length have little impact on the displacement at ground level.
Broms has also provided the following formula for calculating the lateral displacement at ground level, for a long free-head pile under a lateral load:

Poulos Method for Linear Analysis of Piles Under Lateral Loads
Poulos and Davis (1980), in their analysis, considered the soil as an elastic and continuous environment, because the displacements of a point are under the influence of the tensions and forces of other parts of the soil. In this method, the soil is assumed to be a semi-infinite, homogeneous, and isotropic environment with a linear elastic behavior. Poulos in order to calculate the displacement of soil, used Mindlin equation which is for horizontal displacement of the soil under a horizontal load in a semi-infinite environment. He also uses the beam theory for the displacement of piles. In this section, only the final solution is described, by taking the displacements and moments into consideration.
Poulos et al conducted their analyses, for the following two types of soil:
- Uniform soil, with the Young's modulus E-S constant with depth
- Soil, with a linear increase of soil modulus ES with depth
- The relationships Poulus presented, have taken the effect of the yielding of soil on displacement, gradient, and moment into account. However, this item is skipped in this section.
The Youngs Modulus Constant with Depth for a Free-Head Pile
For a soil with perfectly elastic behavior, the displacement at ground level is obtained by the following relations:

Iyp: Impact factor for calculating the displacement of the head of a pile under a lateral load at ground level
Iym : Impact factor for calculating the displacement of the head of a pile under a moment applied at ground level
Isp : Impact factor for calculating the rotation of the head of a pile under a lateral load at ground level
Ism : Impact factor for calculating the rotation of the head of a pile under a moment applied at ground level
and values are obtained from the graph of Fig. 4, 5 and 6. In these graphs, is the flexibility factor, and its value is obtained from the following relation:

The maximum moment in a free-head pile subjected to a lateral load, can be calculated using the figure 5-22. In this graph, the Poisson's ratio is assumed to be equal to 0.5.
 |
Figure 4: The impact factor Iyp Click here to View figure |
 |
Figure 5: The impact factors Iym, Isp Click here to View figure |
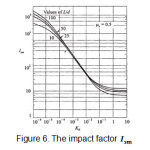 |
Figure 6: The impact factor Ism Click here to View figure |
Analyzing the Non-Linear Behavior of A Long Pile Under A Lateral Load Using the Direct Method
The basis of solving the problems of piles under lateral loads, is obtaining a relation for nh The current relation for nh does not depend on any characteristics of the soil, pile and applied lateral load. This is while, nh is dependent on the relative density of the soil for piles placed in sand, and undrained shear strength for piles placed in clay. nh and the characteristics of the soil and piles is presented by Murthy based on dimensional analyses.
The parameter nh can be expressed as a function of the following parameters, for a pile located in sand and clay:
Piles located in sand nh = ƒs (EI, d, Pe, y, Φ)
Piles located in sand nh = ƒc (EI, d, Pe, y, C)
is a load applied to a pile at ground level, and is used instead of the parameter which is applied at the height of e from the ground level, and is obtained from the following relation:

Based on the dimensional analysis, the following two dimensionless parameters are obtained in the sand and clay:
Piles in sand

In these relations, CΦ is a correction factor for the friction angle Φ , and is obtained from the following relation:

Piles in Clay
Dimensionless parameters for this mode are:
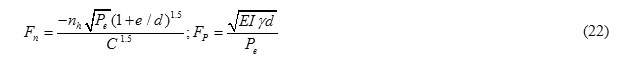
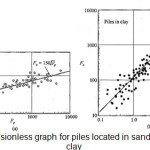
By a set of data obtained from experiments, the graph ∫Fn based on Fp, is drawn as in Figure 7.
 |
|
Problem Definition
In this section, a steel-bar pile with a length of 20 m, an external diameter of 61 cm and a thickness of 2.5 cm, which is embedded in a layer of loose saturated sand with a relative density of 30%, is analyzed using difference equation, non-dimensional, Broms, Poulos, and the direct methods and numerical analysis, and the amount of displacement of the head of the pile under the lateral load of 268 kN/m2, will be presented. Submerged specific gravity of this sand is 8.75 , and its internal friction angle is 33 degrees. The EI of the pile is equal to 4.35 1011 kg-cm2 .
Using the methods described in the previous sections, this pile was analyzed and the results are shown in table 1. In this article, the finite element software Plaxis 3D is used to calculate the lateral displacement at ground level. The way of lateral displacement in the intended pile is as in Figure 8.
Table 1: The values of the lateral displacement of a pile under a lateral load by using different methods.
|
The amount of displacement (cm) |
The calculation method |
|
2.33 |
Difference equation |
|
1.96 |
Non-dimensional |
|
1.4 |
Broms |
|
4.58 |
Poulos |
|
3.77 |
Direct |
|
6.76 |
Numerical |
 |
Figure 8: The graph of horizontal displacement at selected points, and the way of horizontal displacement of the element of the pile under the lateral load Click here to View figure |
Conclusion
Analytical model, in fact, is the representative and replacement of the fundamental aspects of a system for describing its behavior, in a functional and applicable way. In an analytical model, emphasizing the fundamental aspects on the one hand, and maintaining the simplicity on the other hand, are necessary for idealization to be done. Idealization means the use of simplifying assumptions. Even though these simplifying assumptions are false, but it is hoped that they do not affect the model answer. Thus, any analytical model faces a kind of error or approximation.
Answers obtained from analytical models, because of the simplifications which are made, can be very different from the reality. Thus checking the validity of the results of any analytical model is an important part of researches on analytical models.
According to the results obtained from manual analysis and numerical analysis methods, it can be said that the lateral displacement, in terms of ranking, has been calculated greater in Poulos manual method than in all other methods, and has been calculated less in Broms method than in other methods. As it is obvious, the amount of displacement obtained from the three-dimensional software Plaxis, is greater than the values obtained from the manual analysis. The reason the amount of displacement obtained from the software is greater than the results obtained from the manual methods, is that in the numerical analysis, the behavior of materials is assumed to be elastoplastic and the plastic behavior of the soil is also taken into account. This is while, in manual methods, due to the simplifications for analysis, the type of the soil behavior will not be taken into consideration.
It seems that the analytical methods for piles under lateral loads, can no longer be appropriate methods for the analysis, due to the expansion of numerical modeling software programs. Because the accuracy of these methods is very low for solving complex problems with different boundary conditions and non-homogeneous materials, and in case of complexity, these methods will become inefficient.
References
- Broms, B. (1964a).The Lateral Resistance of piles in cohesive soils. Journal of Soil Mechanics & Foundation Division, ASCE, 90 (SM2), 27-63.
- Broms, B. (1964b).The lateral resistance of piles in cohesive soils Journal of Soil Mechanics & Foundation Division, ASCE, 90 (SM3), 123-56.
- Palmer, L.A. and Thompson, J.B. (1948). The earth pressure and deflection along the embedded lengths of piles subjected to lateral thrusts. Proceedings of 2nd Int. Conf. on Soil Mechanics and Foundation Engineering, Rotterdam, 5, 156-161.
- Murthy, (2007).''Advanced Foundation Engineering'', Forth Ed., Consulting Geotechnical Engineer Bangalor, India.
- Reese, L.C. and Matlock, H. (1956). Non-dimensional solutions for laterally-loaded piles with soil modulus assumed proportional to depth. Proceedings of the 8th Texas Conference on Soil Mechanics and Foundation Engineering, Austin, Texas, pp.1-41.
- Vesic, AS (1963).''Bearing Capacity of Deep Foundation in Sand'', Highway Research Record, No. 39, Highway Research Board, Washington DC.
- Vesic, AS (1963).''Discussion-Session III'', Proc. Of the 1st. Int. Conf. on Structural Design of Asphalt Pavements, University of Michigau.
- Vesic, AS (1964). ''Investigation of Bearing Capacity of Pile in sand'', Proc. No. Amer. Conf. on Deep foundations, Mexico city, Vol. 1.
- Vesic, AS (1967).''A Study of Bearing Capacity of Deep Foundation'', Final Report School of Civil Engg., Georgia Inst. Tech., Atlanta, USA.
- Vesic, AS (1970). ''Tests on Instrumented Pile-Ogeechee River Site'', JSMFD, ASCE, Vol. 96. No. SM 2.







